Advanced Placement (AP), 18.07.2022 14:50 holmesleauja

Solve the following differential equation with initial conditions: y''=e^-2t+10e^4t ; y(0)=1, y'(0)=0

Answers

Option A. 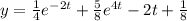$y = \frac{1}{4} e^{-2t} + \frac{5}{8} e^{4t} - 2 t + \frac{1}{8}$

Explanation:

This is a second order DE, so we'll need to integrate twice, applying initial conditions as we go. At a couple points, we'll need to apply u-substitution.

Round 1:

To solve the differential equation, write it as differentials, move the differential, and integrate both sides:

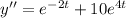
$y''=e^{-2t}+10e^{4t}$

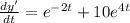
$\frac{dy'}{dt}=e^{-2t}+10e^{4t}$

$dy'=[e^{-2t}+10e^{4t}]dt$

$\int dy'=\int [e^{-2t}+10e^{4t}]dt$

Applying various properties of integration:

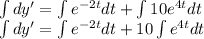
$\int dy'=\int e^{-2t} dt + \int 10e^{4t}dt\\\int dy'=\int e^{-2t} dt + 10\int e^{4t}dt$

Prepare for integration by u-substitution

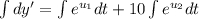$\int dy'=\int e^{u_1} dt + 10\int e^{u_2}dt$ , letting 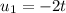u_1=-2t and u_2=4t

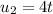
Find dt in terms of 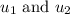$u_1 \text{ and } u_2$

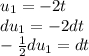
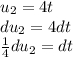
$u_1=-2t\\du_1=-2dt\\-\frac{1}{2}du_1=dt$ $u_2=4t\\du_2=4dt\\\frac{1}{4}du_2=dt$

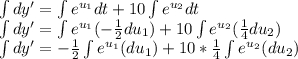
$\int dy'=\int e^{u_1} dt + 10\int e^{u_2}dt\\\int dy'=\int e^{u_1} (-\frac{1}{2} du_1) + 10\int e^{u_2} (\frac{1}{4} du_2)\\\int dy'=-\frac{1}{2} \int e^{u_1} (du_1) + 10 *\frac{1}{4} \int e^{u_2} (du_2)$

Using the Exponential rule (don't forget your constant of integration):

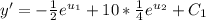
$y'=-\frac{1}{2} e^{u_1} + 10 *\frac{1}{4}e^{u_2} +C_1$

Back substituting for 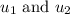$u_1 \text{ and } u_2$ :

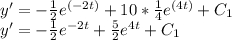
$y'=-\frac{1}{2} e^{(-2t)} + 10 *\frac{1}{4}e^{(4t)} +C_1\\y'=-\frac{1}{2} e^{-2t} + \frac{5}{2}e^{4t} +C_1\\$

Finding the constant of integration

Given initial condition 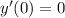y'(0)=0

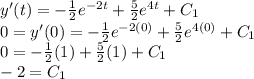
$y'(t)=-\frac{1}{2} e^{-2t} + \frac{5}{2}e^{4t} +C_1\\0=y'(0)=-\frac{1}{2} e^{-2(0)} + \frac{5}{2}e^{4(0)} +C_1\\0=-\frac{1}{2} (1) + \frac{5}{2}(1) +C_1\\-2=C_1\\$

The first derivative with the initial condition applied: 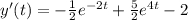$y'(t)=-\frac{1}{2} e^{-2t} + \frac{5}{2}e^{4t} -2\\$

Round 2:

Integrate again:

$y' =-\frac{1}{2} e^{-2t} + \frac{5}{2}e^{4t} -2\\\frac{dy}{dt} =-\frac{1}{2} e^{-2t} + \frac{5}{2}e^{4t} -2\\dy =[-\frac{1}{2} e^{-2t} + \frac{5}{2}e^{4t} -2]dt\\\int dy =\int [-\frac{1}{2} e^{-2t} + \frac{5}{2}e^{4t} -2]dt\\\int dy =\int -\frac{1}{2} e^{-2t} dt + \int \frac{5}{2}e^{4t} dt - \int 2 dt\\\int dy = -\frac{1}{2} \int e^{-2t} dt + \frac{5}{2} \int e^{4t} dt - 2 \int dt\\$

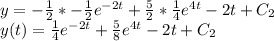
$y = -\frac{1}{2} * -\frac{1}{2} e^{-2t} + \frac{5}{2} * \frac{1}{4} e^{4t} - 2 t + C_2\\y(t) = \frac{1}{4} e^{-2t} + \frac{5}{8} e^{4t} - 2 t + C_2$

Finding the constant of integration :

Given initial condition 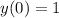y(0)=1

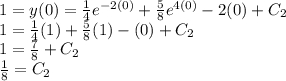
$1=y(0) = \frac{1}{4} e^{-2(0)} + \frac{5}{8} e^{4(0)} - 2 (0) + C_2\\1 = \frac{1}{4} (1) + \frac{5}{8} (1) - (0) + C_2\\1 = \frac{7}{8} + C_2\\\frac{1}{8}=C_2$

So, 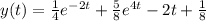$y(t) = \frac{1}{4} e^{-2t} + \frac{5}{8} e^{4t} - 2 t + \frac{1}{8}$

Checking the solution

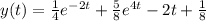
$y(t) = \frac{1}{4} e^{-2t} + \frac{5}{8} e^{4t} - 2 t + \frac{1}{8}$

This matches our initial conditions here 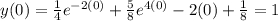$y(0) = \frac{1}{4} e^{-2(0)} + \frac{5}{8} e^{4(0)} - 2 (0) + \frac{1}{8} = 1$

Going back to the function, differentiate:

$y' = [\frac{1}{4} e^{-2t} + \frac{5}{8} e^{4t} - 2 t + \frac{1}{8}]'\\y' = [\frac{1}{4} e^{-2t}]' + [\frac{5}{8} e^{4t}]' - [2 t]' + [\frac{1}{8}]'\\y' = \frac{1}{4} [e^{-2t}]' + \frac{5}{8} [e^{4t}]' - 2 [t]' + [\frac{1}{8}]'$

Apply Exponential rule and chain rule, then power rule

$y' = \frac{1}{4} e^{-2t}[-2t]' + \frac{5}{8} e^{4t}[4t]' - 2 [t]' + [\frac{1}{8}]'\\y' = \frac{1}{4} e^{-2t}(-2) + \frac{5}{8} e^{4t}(4) - 2 (1) + (0)\\y' = -\frac{1}{2} e^{-2t} + \frac{5}{2} e^{4t} - 2$

This matches our first order step and the initial conditions there.

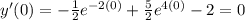
$y'(0) = -\frac{1}{2} e^{-2(0)} + \frac{5}{2} e^{4(0)} - 2=0$

Going back to the function y', differentiate:

$y' = -\frac{1}{2} e^{-2t} + \frac{5}{2} e^{4t} - 2\\y'' = [-\frac{1}{2} e^{-2t} + \frac{5}{2} e^{4t} - 2]'\\y'' = [-\frac{1}{2} e^{-2t}]' + [\frac{5}{2} e^{4t}]' - [2]'\\y'' = -\frac{1}{2} [e^{-2t}]' + \frac{5}{2} [e^{4t}]' - [2]'$

Applying the Exponential rule and chain rule, then power rule

$y'' = -\frac{1}{2} e^{-2t}[-2t]' + \frac{5}{2} e^{4t}[4t]' - [2]'\\y'' = -\frac{1}{2} e^{-2t}(-2) + \frac{5}{2} e^{4t}(4) - (0)\\y'' = e^{-2t} + 10 e^{4t}$

So our proposed solution is a solution to the differential equation, and satisfies the initial conditions given.

Answer from: Quest

answer: the net deposit amount (option c)

in order to fill in the deposit slip, michael needs to fill in the amount that he wants to deposit in the bank. without the net deposit amount, he will not be able to deposit the money because it is mandatory for him to mention it, as the bank needs to maintain a record to avoid any inconveniences for the bank and michael in the future.

the bank officer checks and verifies the amount received against the amount written on the deposit slip to process the slip.

Answer from: Quest

The outer ear canal and it protects you from getting bad infections. hope this xx

Other questions on the subject: Advanced Placement (AP)

Advanced Placement (AP), 24.06.2019 06:00, jarryd4202

Analyze and evaluate the motivation and the rationale behind western expansion through the louisiana purchase that took hold in the united states at the start of the 19th century (long essay question)

Answers: 3

continue

Advanced Placement (AP), 25.06.2019 09:10, s924704

Describe one of your personality traits that you believe to be highly heritable and another trait that seems to be much less so. provide reasons for your answer, and explain why you would expect genetics to exert a greater impact on some personality traits than on others. 10. discuss at least 3 cultural/environmental factors that may influence behavior.

Answers: 3

continue

Advanced Placement (AP), 25.06.2019 13:30, corcor19

Arandom variable x has a mean of 10 and a standard deviation of 3. if each value of x is multiplied by 2, what will the new mean and standard deviation be?

Answers: 1

continue

Advanced Placement (AP), 26.06.2019 00:30, nolimitsteve3210

What is the american psychological association’s ethical principle of psychologists and code of conduct based upon? a. the work of a dozen world-renowned philosophers and ethical savants. b. the feedback of thousands of psychologists and their ethical struggles. c. the regulatory power and authority of the united states constitution. d. the hippocratic rules and guidelines of medical practitioners and surgeons. select the best answer from the choices provided a b c

Answers: 1

continue

You know the right answer?

Solve the following differential equation with initial conditions: y''=e^-2t+10e^4t ; y(0)=1, y'(0)=...